Did the Early Cosmos Inflate Like a Balloon? A Mirror Universe Going Backwards in Time May Be a Simpler Explanation
We live in a golden age for learning about the universe. Our most powerful telescopes have revealed that the cosmos is surprisingly simple on the largest visible scales. Likewise, our most powerful “microscope,” the Large Hadron Collider, has found no deviations from known physics on the tiniest scales.
These findings were not what most theorists expected. Today, the dominant theoretical approach combines string theory, a powerful mathematical framework with no successful physical predictions as yet, and “cosmic inflation”—the idea that, at a very early stage, the universe ballooned wildly in size. In combination, string theory and inflation predict the cosmos to be incredibly complex on tiny scales and completely chaotic on very large scales.
The nature of the expected complexity could take a bewildering variety of forms. On this basis, and despite the absence of observational evidence, many theorists promote the idea of a “multiverse”: an uncontrolled and unpredictable cosmos consisting of many universes, each with totally different physical properties and laws.
So far, the observations indicate exactly the opposite. What should we make of the discrepancy? One possibility is that the apparent simplicity of the universe is merely an accident of the limited range of scales we can probe today, and that when observations and experiments reach small enough or large enough scales, the asserted complexity will be revealed.
The other possibility is that the universe really is very simple and predictable on both the largest and smallest scales. I believe this possibility should be taken far more seriously. For, if it is true, we may be closer than we imagined to understanding the universe’s most basic puzzles. And some of the answers may already be staring us in the face.
The Trouble With String Theory and Inflation
The current orthodoxy is the culmination of decades of effort by thousands of serious theorists. According to string theory, the basic building blocks of the universe are minuscule, vibrating loops and pieces of sub-atomic string. As currently understood, the theory only works if there are more dimensions of space than the three we experience. So, string theorists assume that the reason we don’t detect them is that they are tiny and curled up.
Unfortunately, this makes string theory hard to test, since there are an almost unimaginable number of ways in which the small dimensions can be curled up, with each giving a different set of physical laws in the remaining, large dimensions.
Meanwhile, cosmic inflation is a scenario proposed in the 1980s to explain why the universe is so smooth and flat on the largest scales we can see. The idea is that the infant universe was small and lumpy, but an extreme burst of ultra-rapid expansion blew it up vastly in size, smoothing it out and flattening it to be consistent with what we see today.
Inflation is also popular because it potentially explains why the energy density in the early universe varied slightly from place to place. This is important because the denser regions would have later collapsed under their own gravity, seeding the formation of galaxies.
Over the past three decades, the density variations have been measured more and more accurately both by mapping the cosmic microwave background—the radiation from the big bang—and by mapping the three-dimensional distribution of galaxies.
In most models of inflation, the early extreme burst of expansion which smoothed and flattened the universe also generated long-wavelength gravitational waves—ripples in the fabric of space-time. Such waves, if observed, would be a “smoking gun” signal confirming that inflation actually took place. However, so far the observations have failed to detect any such signal. Instead, as the experiments have steadily improved, more and more models of inflation have been ruled out.
Furthermore, during inflation, different regions of space can experience very different amounts of expansion. On very large scales, this produces a multiverse of post-inflationary universes, each with different physical properties.
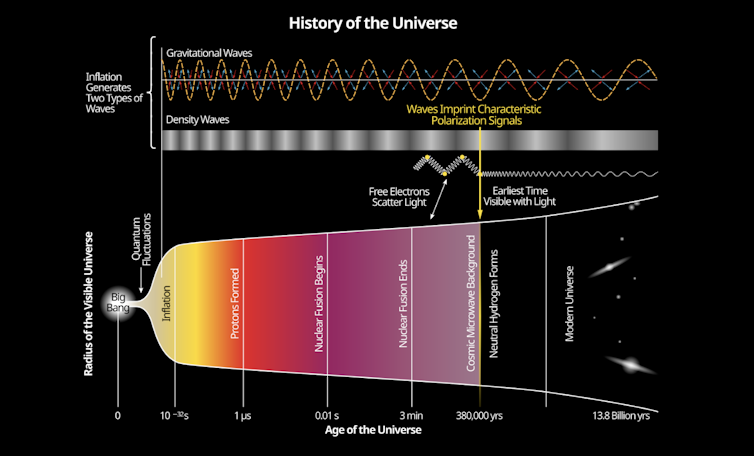
The history of the universe according to the model of cosmic inflation. Image Credit: Wikipedia, CC BY-SA
The inflation scenario is based on assumptions about the forms of energy present and the initial conditions. While these assumptions solve some puzzles, they create others. String and inflation theorists hope that somewhere in the vast inflationary multiverse, a region of space and time exists with just the right properties to match the universe we see.
However, even if this is true (and not one such model has yet been found), a fair comparison of theories should include an “Occam factor,” quantifying Occam’s razor, which penalizes theories with many parameters and possibilities over simpler and more predictive ones. Ignoring the Occam factor amounts to assuming that there is no alternative to the complex, unpredictive hypothesis—a claim I believe has little foundation.
Over the past several decades, there have been many opportunities for experiments and observations to reveal specific signals of string theory or inflation. But none have been seen. Again and again, the observations turned out simpler and more minimal than anticipated.
It is high time, I believe, to acknowledge and learn from these failures and to start looking seriously for better alternatives.
A Simpler Alternative
Recently, my colleague Latham Boyle and I have tried to build simpler and more testable theories that do away with inflation and string theory. Taking our cue from the observations, we have attempted to tackle some of the most profound cosmic puzzles with a bare minimum of theoretical assumptions.
Our first attempts succeeded beyond our most optimistic hopes. Time will tell whether they survive further scrutiny. However, the progress we have already made convinces me that, in all likelihood, there are alternatives to the standard orthodoxy—which has become a straitjacket we need to break out of.
I hope our experience encourages others, especially younger researchers, to explore novel approaches guided strongly by the simplicity of the observations—and to be more skeptical about their elders’ preconceptions. Ultimately, we must learn from the universe and adapt our theories to it rather than vice versa.
Boyle and I started out by tackling one of cosmology’s greatest paradoxes. If we follow the expanding universe backward in time, using Einstein’s theory of gravity and the known laws of physics, space shrinks away to a single point, the “initial singularity.”
In trying to make sense of this infinitely dense, hot beginning, theorists including Nobel laureate Roger Penrose pointed to a deep symmetry in the basic laws governing light and massless particles. This symmetry, called “conformal” symmetry, means that neither light nor massless particles actually experience the shrinking away of space at the big bang.
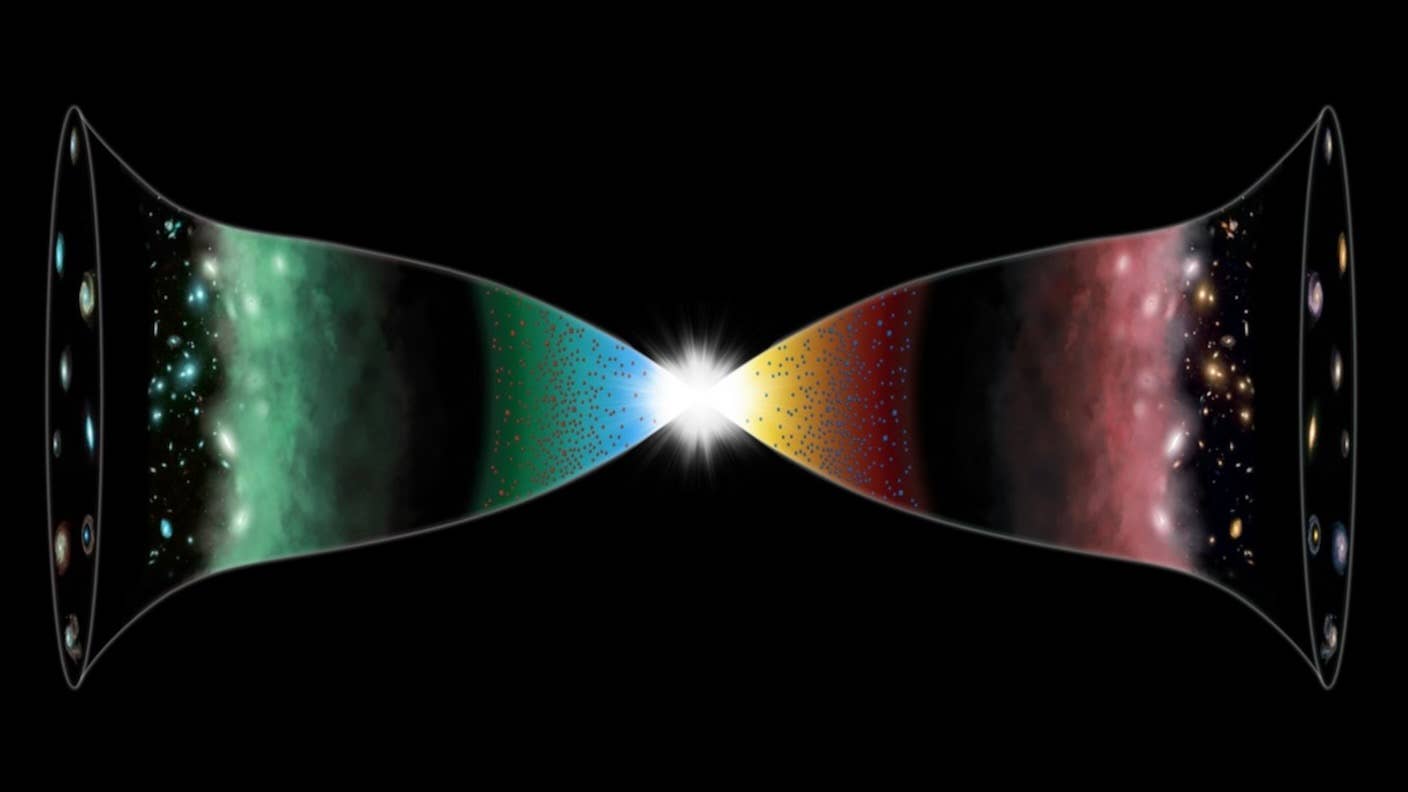
By exploiting this symmetry, one can follow light and particles all the way back to the beginning. Doing so, Boyle and I found we could describe the initial singularity as a “mirror”: a reflecting boundary in time (with time moving forward on one side, and backward on the other).
Picturing the big bang as a mirror neatly explains many features of the universe which might otherwise appear to conflict with the most basic laws of physics. For example, for every physical process, quantum theory allows a “mirror” process in which space is inverted, time is reversed, and every particle is replaced with its anti-particle (a particle similar to it in almost all respects, but with the opposite electric charge).
Be Part of the Future
Sign up to receive top stories about groundbreaking technologies and visionary thinkers from SingularityHub.


According to this powerful symmetry, called CPT symmetry, the “mirror” process should occur at precisely the same rate as the original one. One of the most basic puzzles about the universe is that it appears to violate CPT symmetry because time always runs forward and there are more particles than anti-particles.
Our mirror hypothesis restores the symmetry of the universe. When you look in a mirror, you see your mirror image behind it: if you are left-handed, the image is right-handed and vice versa. The combination of you and your mirror image are more symmetrical than you are alone.
Likewise, when Boyle and I extrapolated our universe back through the big bang, we found its mirror image, a pre-bang universe in which (relative to us) time runs backward and antiparticles outnumber particles. For this picture to be true, we don’t need the mirror universe to be real in the classical sense (just as your image in a mirror isn’t real). Quantum theory, which rules the microcosmos of atoms and particles, challenges our intuition so at this point the best we can do is think of the mirror universe as a mathematical device which ensures that the initial condition for the universe does not violate CPT symmetry.
Surprisingly, this new picture provided an important clue to the nature of the unknown cosmic substance called dark matter. Neutrinos are very light, ghostly particles which, typically, move at close to the speed of light and which spin as they move along, like tiny tops. If you point the thumb of your left hand in the direction the neutrino moves, then your four fingers indicate the direction in which it spins. The observed, light neutrinos are called “left-handed” neutrinos.
Heavy “right-handed” neutrinos have never been seen directly, but their existence has been inferred from the observed properties of light, left-handed neutrinos. Stable, right-handed neutrinos would be the perfect candidate for dark matter because they don’t couple to any of the known forces except gravity. Before our work, it was unknown how they might have been produced in the hot early universe.
Our mirror hypothesis allowed us to calculate exactly how many would form and to show they could explain the cosmic dark matter.
A testable prediction followed: If the dark matter consists of stable, right-handed neutrinos, then one of three light neutrinos that we know of must be exactly massless. Remarkably, this prediction is now being tested using observations of the gravitational clustering of matter made by large-scale galaxy surveys.
The Entropy of Universes
Encouraged by this result, we set about tackling another big puzzle: Why is the universe so uniform and spatially flat, not curved, on the largest visible scales? The cosmic inflation scenario was, after all, invented by theorists to solve this problem.
Entropy is a concept which quantifies the number of different ways a physical system can be arranged. For example, if we put some air molecules in a box, the most likely configurations are those which maximize the entropy—with the molecules more or less smoothly spread throughout space and sharing the total energy more or less equally. These kinds of arguments are used in statistical physics, the field which underlies our understanding of heat, work, and thermodynamics.
The late physicist Stephen Hawking and collaborators famously generalized statistical physics to include gravity. Using an elegant argument, they calculated the temperature and the entropy of black holes. Using our “mirror” hypothesis, Boyle and I managed to extend their arguments to cosmology and to calculate the entropy of entire universes.
To our surprise, the universe with the highest entropy (meaning it is the most likely, just like the atoms spread out in the box) is flat and expands at an accelerated rate, just like the real one. So statistical arguments explain why the universe is flat and smooth and has a small positive accelerated expansion, with no need for cosmic inflation.
How would the primordial density variations, usually attributed to inflation, have been generated in our symmetrical mirror universe? Recently, we showed that a specific type of quantum field (a dimension zero field) generates exactly the type of density variations we observe, without inflation. Importantly, these density variations aren’t accompanied by the long wavelength gravitational waves which inflation predicts—and which haven’t been seen.
These results are very encouraging. But more work is needed to show that our new theory is both mathematically sound and physically realistic.
Even if our new theory fails, it has taught us a valuable lesson. There may well be simpler, more powerful and more testable explanations for the basic properties of the universe than those the standard orthodoxy provides.
By facing up to cosmology’s deep puzzles, guided by the observations and exploring directions as yet unexplored, we may be able to lay more secure foundations for both fundamental physics and our understanding of the universe.
This article is republished from The Conversation under a Creative Commons license. Read the original article.
Image Credit: The mirror universe, with the big bang at the center / Neil Turok, CC BY-SA
Neil Turok is the inaugural Higgs Chair of Theoretical Physics at the University of Edinburgh. He holds the Roger Penrose Visiting Professorship at Perimeter Institute for Theoretical Physics in Canada where he is emeritus director. Before that he was Chair of Mathematical Physics at Cambridge and Professor of Physics at Princeton. Neil’s day job is developing and testing fundamental physics theories of the universe. His first-principles predictions of correlations between the temperature and polarization of the cosmic background radiation (CBR) and the CBR and the galaxy distribution have been confirmed at high precision. His work also ruled out several popular theoretical models. Recently, he showed how a CPT-symmetric universe could provide the simplest-yet explanation of the cosmic dark matter, the large scale properties of the universe, and the primordial density variations. Born in South Africa, Neil founded the African Institute for Mathematical Sciences (AIMS), now the continent’s leading institute for postgraduate training and research in the mathematical sciences. AIMS has over 3,500 Masters alumni, from 45 African countries. More than 800 have proceeded to PhDs. In 2008, Neil won the TED prize for his research and for AIMS. In 2016, he was made an Honorary Fellow of the UK Institute of Physics and was awarded the John Torrence Tate Medal of the American Institute of Physics for International Leadership in Physics. In 2018, he was appointed an Officer of the Order of Canada.
What we’re reading